Dhistribusi t-student

Sajeroning probabilitas lan statistika, Distribusi t-student utawa Student’s t-distribution (asring dicekak dadi t-distribution) iku sawijining distribusi probabilitas lumintu (continuous probability distribution sing dianggo nalika nganakaké èstrimasi aji rata-rata (mean) saka sawijining populasi sing ukuran sampelé cilik lan standard déviasi ora diweruhi.

Student’s t

Probability density function
Cumulative distribution function
Parameters	$\nu$ > 0 degrees of freedom (real)
Support	x ∈ (−∞; +∞)
PDF	$\textstyle {\frac {\Gamma \left({\frac {\nu +1}{2}}\right)}{{\sqrt {\nu \pi }}\,\Gamma \left({\frac {\nu }{2}}\right)}}\left(1+{\frac {x^{2}}{\nu }}\right)^{-{\frac {\nu +1}{2}}}\!$
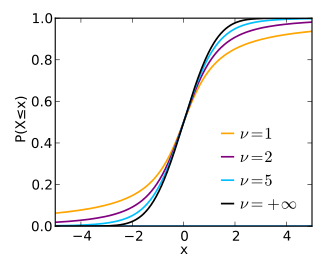
CDF	${\begin{matrix}{\frac {1}{2}}+x\Gamma \left({\frac {\nu +1}{2}}\right)\cdot \\[0.5em]{\frac {\,_{2}F_{1}\left({\frac {1}{2}},{\frac {\nu +1}{2}};{\frac {3}{2}};-{\frac {x^{2}}{\nu }}\right)}{{\sqrt {\pi \nu }}\,\Gamma \left({\frac {\nu }{2}}\right)}}\end{matrix}}$ where 2F1 is the hypergeometric function
Mean	0 for $\nu$ > 1, otherwise undefined
Median	0
Mode	0
Variance	$\textstyle {\frac {\nu }{\nu -2}}$ for $\nu$ > 2, ∞ for 1 < $\nu$ ≤ 2, otherwise undefined
Skewness	0 for $\nu$ > 3
Ex. kurtosis	$\textstyle {\frac {6}{\nu -4}}$ for $\nu$ > 4
Entropy	${\begin{matrix}{\frac {\nu +1}{2}}\left[\psi \left({\frac {1+\nu }{2}}\right)-\psi \left({\frac {\nu }{2}}\right)\right]\\[0.5em]+\log {\left[{\sqrt {\nu }}B\left({\frac {\nu }{2}},{\frac {1}{2}}\right)\right]}\end{matrix}}$ ψ: digamma function, B: beta function
MGF	undefined
CF	$\textstyle {\frac {K_{\nu /2}\left({\sqrt {\nu }}|t|)({\sqrt {\nu }}|t|\right)^{\nu /2}}{\Gamma (\nu /2)2^{\nu /2-1}}}$ for $\nu$ > 0 $K_{\nu }$(x): Bessel function

Dhéfinisi

Fungsi dènsiti probabilitas

Fungsi dhènsitas probabilitas saka distribusi t-Student sing standard ya iku:

$f(t)={\frac {\Gamma ({\frac {\nu +1}{2}})}{{\sqrt {\nu \pi }}\,\Gamma ({\frac {\nu }{2}})}}\left(1+{\frac {t^{2}}{\nu }}\right)^{-{\frac {\nu +1}{2}}},\!$

ing ngendi $\nu$ minangka drajad kabébasan lan $\Gamma$ minangka fungsi Gamma. Bisa uga ditulis:

$f(t)={\frac {1}{{\sqrt {\nu }}\,B\left({\frac {1}{2}},{\frac {\nu }{2}}\right)}}\left(1+{\frac {t^{2}}{\nu }}\right)^{-{\frac {\nu +1}{2}}}\!,$

ing ngendi B iku wujud fungsi Beta.

Kanggo $\nu$ genep,

${\frac {\Gamma ({\frac {\nu +1}{2}})}{{\sqrt {\nu \pi }}\,\Gamma ({\frac {\nu }{2}})}}={\frac {(\nu -1)(\nu -3)\cdots 5\cdot 3}{2{\sqrt {\nu }}(\nu -2)(\nu -4)\cdots 4\cdot 2\,}}.$

Kanggo $\nu$ ganjil,

${\frac {\Gamma ({\frac {\nu +1}{2}})}{{\sqrt {\nu \pi }}\,\Gamma ({\frac {\nu }{2}})}}={\frac {(\nu -1)(\nu -3)\cdots 4\cdot 2}{\pi {\sqrt {\nu }}(\nu -2)(\nu -4)\cdots 5\cdot 3\,}}.\!$

Gambar-gambar iki nuduhaké dhènsitas saka t-distribution tumrap aji $\nu$ sing tansaya mundhak. Dhistribusi normal dituduhaké kanthi garis biru minangka pembandhing. Pirsanana yèn t-distribution (garis abang) dadi luwih cedhak marang dhistribusi normal nalika aji $\nu$ tansaya gedhé.

Density of the t-distribution (red) for 1, 2, 3, 5, 10, and 30 df compared to the standard normal distribution (blue).
Previous plots shown in green.

1 degree of freedom	2 degrees of freedom	3 degrees of freedom
5 degrees of freedom	10 degrees of freedom	30 degrees of freedom

Pranala njaba

• Earliest Known Uses of Some of the Words of Mathematics (S) (Remarks on the history of the term "Student's distribution")